Question

This composite figure is created by placing a sector of a circle on a rectangle. What is the area of this composite figure? Show your work.

Answer 1

area of the figure: 29.92 m²

formula's:

• area of rectangle: length * width

• area of sector: ∅/360 * πr²

solving steps:

area of rectangle + area of sector

5.5 * 4 + 30/360 * π(5.5)²

22 + 7.919

29.92 m²

Answer 2

Calculate the area of the sector and the area of the rectangle separately, then add them together to determine the area of the composite figure.

Rectangle

Area of a rectangle = width × length

= 4 × 5.5

= 22 m²

Sector

`\textsf{area of sector}=(\theta\pi r^2)/(360) \textsf{ (when } \theta \textsf{ is measured in degrees)}`

`=(30 \cdot\pi\cdot5.5^2)/(360)`

`=(121)/(48)\pi \textsf{ m}^2`

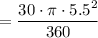
Total area

total area = area of rectangle + area of sector

`=22 +(121)/(48)\pi`

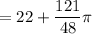
= 29.91943148...

= 29.9 m² (nearest tenth)