Question

1a) Use the − definition of the limit to prove lim→23+4=10.

1b) Use the − definition of the limit to prove lim→4−2=−2.

Answer 1

You're missing symbols in both of your expressions, but considering the first limit has a value of 10, I suspect you meant to write

`\displaystyle \lim_(x\to2)(3x+4) = 10`

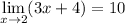
(which is true) but unfortunately I am nowhere near as confident about what the second one is supposed to say. So one proof will have to do, unless you come around to editing your question.

The claim,

`\displaystyle \lim_(x\to2)(3x+4) = 10`

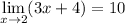
is to say that, for any given ε > 0, we can find δ (a number that depends on ε) such that whenever |x - 2| < δ, this ensures that |(3x + 4) - 10| < ε.

Roughly speaking: if x is close enough to 2, this translates to f(x) = 3x + 4 being close enough to 10. It's our job to figure out how close x needs to be to 2 in order that f(x) is close enough to 10, where the closeness to 10 is some given threshold.

We want to arrive at the inequality,

|(3x + 4) - 10| < ε

so suppose we work backwards. With some simplification and rewriting, we have

|3x - 6| = |3 (x - 2)| = |3| |x - 2| = 3 |x - 2| < ε

and so

|x - 2| < ε/3

which suggests that we should pick δ = ε/3.

Now for the proof itself:

Let ε > 0 be given, and let δ = ε/3. Then

|x - 2| < δ = ε/3

3 |x - 2| < ε

|3x - 6| < ε

|(3x + 4) - 10| < ε

and this completes the proof of the limit. QED