Question

Simplify the expression

Answer 1

`-(4)/(7) -(8)/(7) i√(5)`

Explanation:

To rationalize the denominator, we would have to multiply by the complex conjugate of 6 + 2i√5 which is 6 - 2i√5:

`(8-8i√(5) )/(6+2i√(5) ) *(6-2i√(5) )/(6-2i√(5) )`

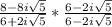
The denominator resembles the difference of squares:

6^2 - (2i√5)^2

36 + 20

56

Next we would need to multiply the numerator, but before, notice we can factor out 8 from 8 - 8i√5:

`(8(1-i√(5))(6-2i√(5)) )/(56)`

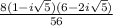
We can cancel that 8 with that 56 in the denominator:

`(6-2i√(5)-6i√(5)-10)/(7)`

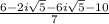
This simplifies to:

`(-4-8i√(5) )/(7)`

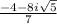
which is the same as:

`-(4)/(7) -(8)/(7) i√(5)`