Question

Need answers, please explain asap! need the answer for 2 and 3!

Answer 1

Key Concepts

Surface Area and Volume of a Cube

Volume of a cube formula:
`V=a^3`

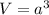
Surface area of a cube formula:
`SA=6a^2`

• 
  `a` is the side length

Area of a Trapezoid

Area of a trapezoid formula:
`A=(1)/(2)(a+b)h`

• 
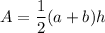
  `a` is the length of the top side
• 
  `b` is the length of the bottom side
• 
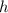  `h` is the height (forms a right angle with the bottom of the trapezoid)

Solving the Question

Question 2

We're given:

• Volume of the cube = 343 cm³
• We must solve for the surface area

First, use the volume formula to solve for the side length:

`V=a^3`

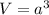
⇒ Plug in the known volume:

`343=a^3\\\sqrt[3]{343}=\sqrt[3]{a^3}\\a=7`

Therefore,
`a=7`.

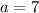Now, plug this value into the formula for surface area:

`SA=6a^2\\SA=6(7)^2\\SA=294`

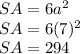
Therefore, the surface area of the cube is 294 cm².

Question 2

We're given:

• One can of paint covers 72 ft²
• 
  `a=14`
• 
  `b=24`
• 
  `h=16.5`

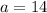
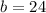
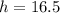
For this question, we must solve for the area of the trapezoid, then divide its double by 72 to find how many cans of paint we need for two coats.

Solve for the area of the trapezoid:

`A=(1)/(2)(a+b)h`

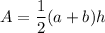
⇒ Plug in the given information:

`A=(1)/(2)(14+24)(16.5)\\\\A=(1)/(2)(38)(16.5)\\\\A=(19)(16.5)\\A=313.5`

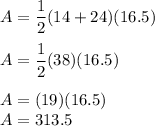
Therefore, the area of the trapezoid is 313.5 ft²

Multiply it by 2:

`313.5*2\\=627`

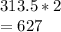
Divide this by 72:

`(627)/(72)\\\\=8.7`

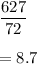
Therefore, we need 9 cans of paint to paint two coats over the deck.