Answer:
Solution :
Verify the following for a = 3 and b = 4.
★ a) (a + b)² = a² + 2ab + b²
Here
Now :-
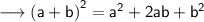
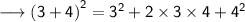
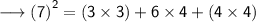
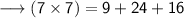
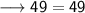

Hence Verified!
━┅━┅━┅━┅━┅━┅━┅━┅━┅━
★ b) (a - b)² = a² - 2ab + b²
Here :-
Now :-
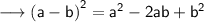

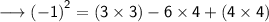
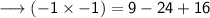
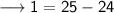


Hence Verified!
━┅━┅━┅━┅━┅━┅━┅━┅━┅━
★ c) (a + b)(a - b) = a² - b²
Here :-
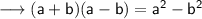
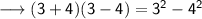
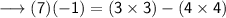
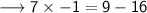
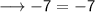

Hence Verified!