Question

HELP ASAP ...........

Answer 1

A

Explanation:

Given

y - 2x - 8 = 0 ( add 2x + 8 to both sides )

y = 2x + 8 → (1)

y² + 8x = 0 → (2)

Substitute y = 2x + 8 into (2)

(2x + 8)² + 8x = 0 ← expand left side using FOIL and simplify

4x² + 32x + 64 + 8x = 0

4x² + 40x + 64 = 0 ( divide through by 4 )

x² + 10x + 16 = 0 ← in standard form

(x + 8)(x + 2) = 0 ← in factored form

x + 8 = 0 ⇒ x = - 8

x + 2 = 0 ⇒ x = - 2

Substitute these values into (1) for corresponding values of y

x = - 8 : y = 2(- 8) + 8 = - 16 + 8 = - 8 ⇒ P (- 8, - 8)

x = - 2 : y = 2(- 2) + 8 = - 4 + 8 = 4 ⇒ Q (- 2, 4 )

Calculate the length of PQ using the distance formula

PQ =
`\sqrt{(x_(2)-x_(1))^2+(y_(2)-y_(1))^2 }`

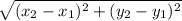
with (x₁, y₁ ) = P (- 8, - 8) and (x₂, y₂ ) = Q (- 2, 4 )

PQ =
`√((-2-(-8))^2+(4-(-8))^2)`

=
`√((-2+8)^2+(4+8)^2)`

=
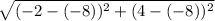
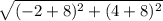
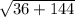
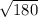
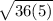
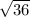`√(6^2+12^2)`

=
`√(36+144)`

=
`√(180)`

=
`√(36(5))`

=
`√(36)` ×
`√(5)`

= 6
`√(5)` → A