Answer:
y + 3 = 10/11(x + 3)
Explanation:
Given the points (-3, -3) and (8, 7), we can use these coordinates to solve for the slope of the line using the formula:
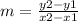
Let (x1, y1) = (-3, -3)
(x2, y2) = (8, 7)
Substitute these values into the slope formula:

Thus, slope (m) = 10/11.
Next, using the slope (m) = 10/11, and one of the given points (-3, -3), we'll substitute these values into the point-slope form:
y - y1 = m(x - x1)
Let (x1, y1) = (-3, -3)
m = 10/11
y - y1 = m(x - x1)
y - (-3) = 10/11[x - (-3)]
Simplify:
y + 3 = 10/11(x + 3) this is the point-slope form.