Question

Find the derivatives.

Answer 1

f ' (0) exists if the limit,

`\displaystyle \lim_(h\to0)\frac{f(0+h)-f(0)}h`

exists, and f ' (0) has the value of this limit.

In order for this limit to exist, both limits from either side of h = 0 must exist.

Suppose h < 0. Then 0 + h is also negative, so by definition of f(x) we have

f (0 + h) = ((0 + h) + 1)² = h ² + 2h + 1

Then in the limit, if h is approaching 0, it must be from below or from the left:

`\displaystyle \lim_(h\to0^-)(f(0+h)-f(0))/(h) = \lim_(h\to0^-)((h^2+2h+1)-1)/(h) = \lim_(h\to0^-)(h+2) = 2`

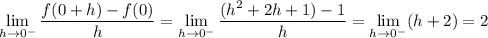
Now suppose h > 0, so that 0 + h is positive and h approaches 0 from above or from the right. Then

f (0 + h) = 2 (0 + h) + 1 = 2h + 1

and

`\displaystyle \lim_(h\to0^+)\frac{f(0+h)-f(0)}h = \lim_(h\to0^+)((2h+1)-1)/(h) = \lim_(h\to0^+)2 = 2`

Both one-sided limits match, so f ' (0) = 2.