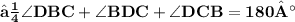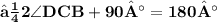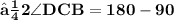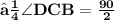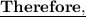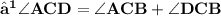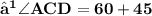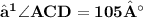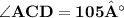
__________________________________________
- BCD is an isosceles right triangle , right angled at D
- ABC is an equilateral triangle

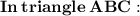
❒ Sum of all angles is 180° , since it is an equilateral triangle all the three angles would be same
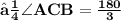
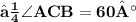

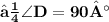
 (Isosceles triangle)
(Isosceles triangle)