Question

Properties of parallelograms. Its one question of an IXL assignment

Answer 1

With the help of the diagram, we can find the value of y.

Solution :

We know that,

• Opposite sides of a parallelogram are equal to each other .

So,

As, opposite sides are equal so,

`\qquad\sf{ \dashrightarrow{ 4y {} = y + 39}}`

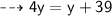
Adding (-y) to both sides :

`\qquad\sf{ \dashrightarrow{ 4y + ( - y) {} = y + ( - y) + 39}}`

`\qquad\sf{ \dashrightarrow{ 3y {} = 39}}`

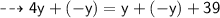
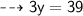
Dividing 3 from both sides :

`\qquad\sf{ \dashrightarrow{ (3y)/(3) {} = (39)/(3) }}`

`\qquad{\pmb{ \bf{ \dashrightarrow{ y {} = 13}} }}`

⠀

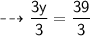
Therefore, y = 13 .

Answer 2

y = 13

Explanation:

According to properties of a parallelogram,

Opposite sides of a parallelogram are equal and parallel so,

4y = y + 39 [Since opposite sides]

=> 4y - y = 39

=> 3y = 39

`= > y = (39)/(3)`

=> y = 13 (Ans)