Use the discriminant to find the number and type of solution for x^2+6x=-9

Question

asked Sep 10, 2022 104k views

1 Answer

Dtanabe · Answer 1 · 2022-09-17T01:55:53+0000

Answer:

D = 0; one real root

Explanation:

Discriminant Formula:

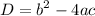
$\displaystyle \large{D = {b}^(2) - 4ac}$

First, arrange the expression or equation in ax^2+bx+c = 0.

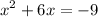
$\displaystyle \large{ {x}^(2) + 6x = - 9}$

Add both sides by 9.

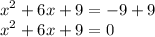
$\displaystyle \large{ {x}^(2) + 6x + 9 = - 9 + 9} \\ \displaystyle \large{ {x}^(2) + 6x + 9 = 0}$

Compare the coefficients so we can substitute in the formula.

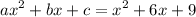
$\displaystyle \large{a {x}^(2) + bx + c = {x}^(2) + 6x + 9 }$

Substitute a = 1, b = 6 and c = 9 in the formula.

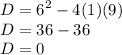
$\displaystyle \large{D = {6}^(2) - 4(1)(9)} \\ \displaystyle \large{D = 36 - 36} \\ \displaystyle \large{D = 0}$

Since D = 0, the type of solution is one real root.