Question

What is an equation of the line that passes through the points (-4, 2) and (-8, -3)? ​

Answer 1

y = 5/4x + 7

Explanation:

Given points (-4, 2) and (-8, -3):

Let (x1, y1) = (-4, 2)

(x2, y2) = (-8, -3)

Use these points to solve for the slope of the line:

`m = (y2 - y1)/(x2 - x1) = (-3 - 2)/(- 8 - (-4)) = (-5)/(-4) = (5)/(4)`

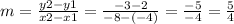
Therefore, the slope of the line is: m = 5/4.

Next, we must determine the y-intercept. In order to do so, we can use the point-slope form and plug in the values of (-4, 2) into the equation as (x1, y1):

y - y1 = m(x - x1)

`y - 2 = (5)/(4)(x - (-4))`

`y - 2 = (5)/(4)(x + 4)`

`y - 2 = (5)/(4)x + 5`

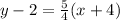
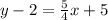
Add 2 on both sides of the equation:

`y - 2 + 2 = (5)/(4)x + 5 + 2`

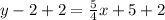
y = 5/4x + 7

Therefore, the equation of the line is: y = 5/4x + 7 where the slope (m) is 5/4, and the y-intercept (b) is 7.