Answer: The answer is: "
 " .
" .
________
Explanation:
________
Given: "
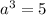 " ; and: "
" ; and: "
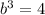 " ;
" ;
Find: "
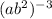 " ;
" ;
________
Note:
"
 ;
;
________
Take note of the following properties of exponents:
_______________________
{Note: "
 ".
".
{Note: "
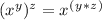 ".
".
{Note: "
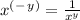 " ; Assuming "
" ; Assuming "
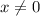 " .}.
" .}.
______________________
We have: "
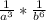 " ;
" ;
________
Given: "
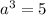 " ; We can substitute:
" ; We can substitute:
________
"
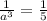 " ;
" ;
________
Now, regarding: "
 " ;
" ;
________
Given: "
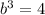 " ;
" ;
Note that " 6 ÷ 3 = 2 " ;
________
So: "
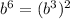 " ; {since: "
" ; {since: "
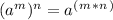 " } ;
" } ;
________
So: We can substitute "4" for "
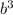 " ;
" ;
and solve for the value of: "
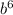 " :
" :
________
"
 " ;
" ;
So: "
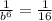 " ;
" ;
________
Now, "
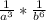 =
=
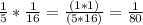 " .
" .
________
The answer is: "
 " .
" .
________
Hope this is helpful to you!
Wishing you the best!
________