
1st Question :-
a) State what's asked to find

- the length of the rectangular garden.
b) State the given facts

- area of a rectangular pool (garden) = x² + x - 12 cm²
- width of the garden = x + 4 cm.
c) Write a working equation

area of the garden = length of the garden × width of the garden. Let's take the length as 'l'. So the equation is...
- x² + x - 12 = l × (x + 4)
d) Solve the equation


e) State your answer

- The length of the rectangular garden is x - 3 cm.
NOTE :-
I think there's a mistake in the question. It should be the area of the rectangular garden & not area of the rectangular pool because here we are asked to measure the length of the garden.
__________________
2nd Question :-
a) State what's asked to find

- the length of the side of the square.
b) State the given facts

- area of the tile = x² + 10x + 25 cm²
c) Write a working equation.
We know that, area of a square = side of the square × side of the square. Let's take the side of the tile (square) as 's'. So, the equation is...
d) Solve the equation

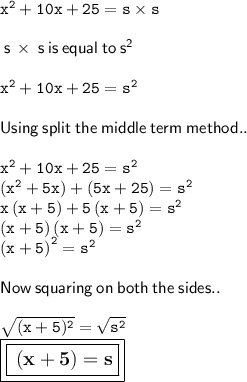
e) State your answer

- The length of 1 side of the square is x + 5 cm.
__________________