Question

Are these shapes similar? Choose all that apply.

No, the corresponding angles are not congruent.


Yes, the corresponding sides are proportional.


Yes, the corresponding angles are all congruent.


No, the corresponding sides are not proportional.

Answer 1

answer is no the corresponding angles are not congruent

Answer 2

the correct choices are A and D. The shapes are not similar.

To determine if the two triangles are similar, we need to compare their corresponding angles and sides. Similar triangles have all corresponding angles equal and all corresponding sides proportional.

Looking at the provided triangles:

For triangle ABC:

- Angle A = 36°

- Angle B = 54°

- Angle C = 90°

- Sides are 12 m, 12 m, and 10 m.

For triangle DEF:

- Angle D = 17°

- Angle E = 90°

- Angle F = 73°

- Sides are 60 m, 40 m, and 30 m.

Corresponding angles must be congruent for two triangles to be similar. In these triangles, only one pair of angles is congruent (the right angles). The other angles in triangle ABC (36° and 54°) do not match the corresponding angles in triangle DEF (17° and 73°), so criterion C is not met.

Now, let's check if the sides are proportional.

For triangle ABC, the ratio of the sides can be given as:

- Side AB to side AC:
`\( (12)/(12) = 1 \)`

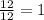
- Side BC to side AC:
`\( (10)/(12) \)`

For triangle DEF, the corresponding ratios would be:

- Side DE to side EF:
`\( (60)/(40) = 1.5 \)`

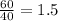
- Side DF to side EF:
`\( (30)/(40) = 0.75 \)`

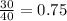
The ratios are not the same; hence, the sides are not proportional, which means criterion B is not met.

Since neither the angles nor the sides are in correspondence, we can conclude:

A. No, the corresponding angles are not congruent.

D. No, the corresponding sides are not proportional.

Therefore, the correct choices are A and D. The shapes are not similar.