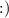1.)
- 0 = 1 occurrence
- 1 = 1 occurrence
- 2 = 1 occurrence
- 3 = 1 occurrence
- 4 = 1 occurrence
- 5 = 0 occurrence
- 6 = 1 occurrence
- 7 = 1 occurrence
- 8 = 1 occurrence
- 9 = 2 occurrences
- 10 = 0 occurrence
- 11 = 2 occurrences
- 12 = 1 occurrence
- 13 = 2 occurrences
- 14 = 0 occurrence
- 15 = 1 occurrence
- 16 = 0 occurrence
- 17 = 1 occurrence
- 18 = 1 occurrence
- 19 = 0 occurrence
- 20 = 2 occurrences
2.)
a. P(even number) = 9/20
b. P(odd number) = 11/20
c. P(greater than or equal to 5) = 15/20 or 3/4
d. P(multiple of 4) = 5/20 or 1/4
e. P(divisible by 3) = 7/20
Explanation:
In Mathematics, probability is a prediction of how howlikely events are to happen. To solve for the probability of an event, count the favorable outcomes of that specific event along with the total number of outcomes. Then, write them down in fraction form. If possible, simplify the fraction in lowest terms. Once you have the answer, you may also convert it to decimal or percent.
P(c) = number of fariable outcomes/total number of outcomes
For this particular problem, a spinner with numbers from 1-20 was spun twenty times. The results are as follows.
[11] [12] [20] [20] [7] [11] [15] [9] [8] [18] [O] [4] [6] [13] [17] (2] [1] [3] [9] [13]
To determine the number of occurrences for each number, arrange the results in ascending order (least to greatest) and count how many each of them has showed.
[O] [1] 12] [3] [41 [6] [7] [8] [9] 19] [11] [11] [12] [13] [13] [15] [17] [18] [20] [20]
- 0 = 1 occurrence
- 1 = 1 occurrence
- 2 = 1 occurrence
- 3 = 1 occurrence
- 4 = 1 occurrence
- 5 = 0 occurrence
- 6 = 1 occurrence
- 7 = 1 occurrence
- 8 = 1 occurrence
- 9 = 2 Occurrences
- 10 = 0 occurrence
- 11 = 2 occurrences
- 12 = 1 occurrence
- 13 = 2 occurrences
- 14 = 0 occurrence
- 15 = 1 occurrence
- 16 = 0 occurrence
- 17 = 1 occurrence
- 18 = 1 occurrence
- 19 = 0 occurrence
- 20 = 2 occurrence
To find the experimental probability of an event, use the formula above. Take note that when countingfor the number of favorable outcomes, even if some numbers are recurring, each of it is still counted.
Among the results, 9 of them are even numbers which are 0, 2,4, 6, 8, 12, 18, 20, and 20 thus, 9 is the number of favorable outcomes while 20 is the number of total outcomes. The experimental probability of spinning an even number is 9/20.
As for the odd numbers, there are 11 which are 1,3, 7,9,9, 11, 11, 13, 13, 15, and 17 thus, 11 is the number of favorable outcomes while 20 is the number of total outcomes. The experimental probability of spinning an even number is 11/20.
For numbers greater than or equal to 5, fifteen of the results are greater than 5. They are 6,7, 8, 9, 9, 11,11, 12, 13, 13, 15, 17, 18, 20, and 20 therefore, the experimental probability of spinning a numbergreater than or equal to 5 is 15/20 or 3/4 in lowestterms. Meanwhile, five of the results are multiples of 4.
They are 4, 8, 12, 20, and 20 therefore, the experimental probability of spinning a number thatis a multiple of 4 is 5/20 or 1/4 in lowest terms.
Last, numbers that are divisible which showed in the results are 3, 6,9, 9, 12, 15, and 18.The probability of spinning a number that is divisible by3 is 7/20.