Answer:
m < 1 = 168°
m < 2 = 12°
Explanation:
Given that the two angles are supplementary, whose sum add up to 180°, and that:
m < 1 = 7x°
m < 2 = (x - 12)°
We can establish the following equation to solve for the measurement of angles 1 and 2:
180° = m < 1 + m < 2
Substitute the values for m < 1 and m < 2 into the equation:
180° = 7x° + (x - 12)°
180° = 7x + x - 12
180° = 8x - 12
Add 12 to both sides:
180° +12 = 8x - 12 + 12
192 = 8x
Divide both sides by 8:
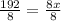
24° = x
Therefore:
m < 1 = 7x° = 7(24)° = 168°
m < 2 = (x - 12)° = (24 - 12)° = 12°
To verify that this is corret, add m < 1 and m < 2:
180° = m < 1 + m < 2
180° = 168° + 12°
Since the measurements of m < 1 and m < 2 add up to 180°, then it means that we derived the correct answers.