Question

How many of the triple permutations of the set A = {a,b,c,d,e} contain e?

PLS NO BOTS​

Answer 1

If one of the elements of a triplet of A must be e, then you have

`\dbinom 42 = (4!)/(2!(4-2)!) = 6`

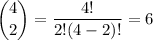
possible choices for the other two elements in the triplet. They are

{a, b, e}

{a, c, e}

{a, d, e}

{b, c, e}

{b, d, e}

{c, d, e}

Multiply this by 3! to account for all possible permutations of a given triplet and you end up with 3! • 6 = 36 possible permutations. They are

abe, aeb, bae, bea, eab, eba

ace, aec, cae, cea, eac, eca

and so on.