
- An isosceles triangle ABC
- AD is a bisector of ∠A
__________________________________________
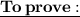
__________________________________________
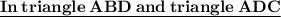

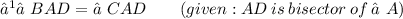
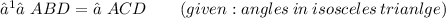
- By ASA criteria ∆ABD congurant to ∆ADC
__________________________________________
- By CPCT ( corresponding parts of congurant triangle )
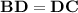
__________________________________________
 Slide to view full answer.
Slide to view full answer.