Answer:
d = 19.796m
Step-by-step explanation:
Since the ball is in the air for 4.02 seconds, the ball should reach the maximum point from the ground in half the total time, therefore, t=2.01s to reach maximum height. At the maximum height, the velocity in the y-direction is 0.
So we know t=2.01, vi=0, g=a=9.8m/s and we are solving for d.
Next, you look for a kinematic equation that has these parameters and the one you should choose is:
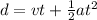
Now by substituting values in, we get
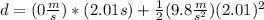
d = 19.796m