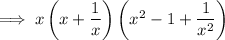Answer:
Question:
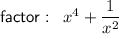
Rewrite the expression using the exponent rule
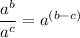
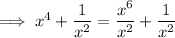
Factor out common term
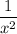 :
:
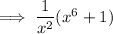
Factor
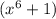 :
:
Apply the exponent rule
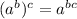 to get cubic exponents:
to get cubic exponents:
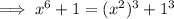
Apply the sum of cubes formula:
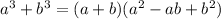
where
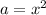 and
and
 :
:

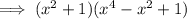
Therefore,
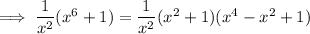
Simplify by dividing the 2nd parentheses by
 :
:
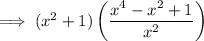
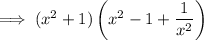
Factor out
 from first parentheses:
from first parentheses: