Answer:
Question (5):
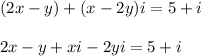
• group imaginary terms and real terms on different sides:
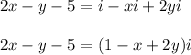
• To find value of x, Imaginary part is zero
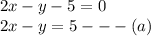
• For Imaginary part, real part is zero:

• solving simultaneously:

Question (4):
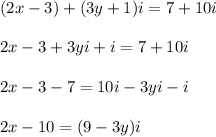
• Group imaginary part and real part
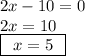
for y:
