Question

The equation is __ ( type your answer in standard form)

Answer 1

First, find the slope of the line between the two points using

`m = (y_2-y_1)/(x_2-x_1)`

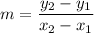
Then use one point to calculate the b for
`y=mx+b`.

Step 1: slope

`\begin{aligned}m&=(7-4)/(-4-2)\\[0.5em]&=(3)/(-6)\\[0.5em]&=-(1)/(2)\end{aligned}`

Step 2: calculate b

Right now we have
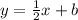`y=(1)/(2)x+b`, so we'll plug in (2,4) for the x and y:

`\begin{aligned}\\4&=-(1)/(2)(2)+b\\[0.5em]4&=-1+b\\[0.5em]5&=b\end{aligned}`

Now we build our slope-intercept equation:
`y=-(1)/(2)x+5`

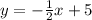
But, this isn't standard form. We need to clear out the fraction and then move the x-term to the left.

Can you take it from there?

Answer 2

In standard form, the answer should be : 2y + x = 6.

Explanation:

See attached image.