Answer:
Explanation:
The absolute value function multiplies its argument by -1 if and only if that argument is negative.
a)

The first condition resolves to ...
x -120 < 0 ⇒ x < 120 . . . . . matches the given condition x < -120
So, we can simplify the expression to ...
-(x -120) = 120 -x . . . when x < -120
__
b)
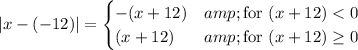
The second condition resolves to ...
x+12 ≥ 0 ⇒ x ≥ -12 . . . . . matches the given condition x > -12
So, we can simplify the expression to ...
x +12 . . . when x > -12
_____
Additional comment
You may have noticed that the function |x -120| translates the absolute value function 120 units to the right. The condition x < -120 puts the boundary at 120 unit to the left of 0, well within the area to the left of the vertex. This is illustrated by the attached graph.