According to the rational root theorem, if a polynomial

has any rational roots, they take the form of ± (some divisor of
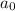 , the constant term) divided by (some divisor of
, the constant term) divided by (some divisor of
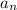 , the coefficient of the leading term).
, the coefficient of the leading term).
In this case, we have
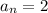 and
and
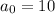 , which have divisors
, which have divisors
• 2: 1, 2
• 10: 1, 2, 5, 10
Then the possible candidates for rational roots are
±1/1 = ±1
±2/1 = ±2
±5/1 = ±5
±10/1 = ±10
±1/2
±2/2 = ±1 (already accounted for)
±5/2
±10/2 = ±5 (already accounted for)