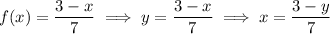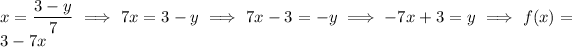Answer:
D
Explanation:
Recall that for a function
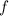 such that
such that
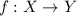 , where
, where
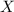 is the Domain and
is the Domain and
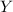 is the Codomain, the function has a inverse, if and only if,
is the Codomain, the function has a inverse, if and only if,
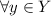 exist an unique
exist an unique
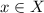 such that
such that
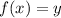 .
.
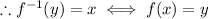
Therefore, the inverse of
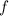 for
for
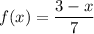 is
is
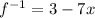
==============================================