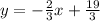Question

LOOK AT IMAGE WORTH 20 points!!!

Write the equation of the line perpendicular to y= 2x+4 that passes through the point ( - 4,9).

Answer 1

`y=-(2)/(3)x+(19)/(3)`

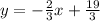
Explanation:

Lines that are perpendicular to each other have opposite reciprocal slopes. So, in this case, the slope that is perpendicular to 3/2 would be -2/3. In order for the line with that slope to pass (-4,9), we would need to plug in the coordinate point and solve for the y-intercept:

`y=mx+b`

`y=-(2)/(3)x+b`

`9=-(2)/(3)(-4)+b`

`9=(8)/(3)+b`

`(19)/(3)=b`

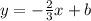
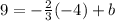
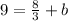
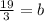
Therefore, the equation in slope-intercept form would be
`y=-(2)/(3)x+(19)/(3)`