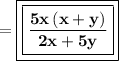- How do you simplify this?
- x²y+xy² / y²+2/5 × xy
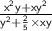
Factor the expressions that are not already factored.
_____
How to factorise :-
NUMERATOR

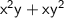
Factor out xy.
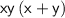
DENOMINATOR

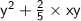
Factor out 1/5.
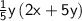
_____
Continuing...
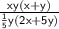
Cancel out y in both the numerator and denominator.
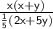
Expand the expression.
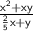
This can further simplified to as