Answer:

Explanation:
Hi there!
Linear equations are typically organized in slope-intercept form:
 where m is the slope and b is the y-intercept (the value of y when x=0)
where m is the slope and b is the y-intercept (the value of y when x=0)
1) Determine the slope (m)
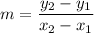 where two points that fall on the line are
where two points that fall on the line are
 and
and

Plug in the points (2,1) and (5,-8):
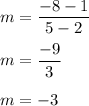
Therefore, the slope of the line is -3. Plug this into
 as m:
as m:
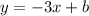
2) Determine the y-intercept (b)
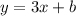
Plug in one of the given points and solve for b:

Therefore, the y-intercept is 7. Plug this back into
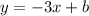 :
:

I hope this helps!