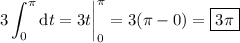I believe I've addressed (1) in another question of yours (24529718).
For (2), the arc length of the curve parameterized by x(t) = 3 cos(t ) and y(t) = 3 sin(t ) over 0 ≤ t ≤ π is
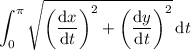
We have
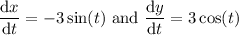
so that the integral reduces to
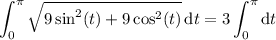
since
 for all t. The remaining integral is trivial:
for all t. The remaining integral is trivial: