Given set ⤵️
73, 68, 73, 85, 92, 81, 88, 35, 48
Mean ↯

- Total number of observations = 9
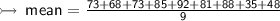
To make the calculation easier, first add the numbers that sum to multiples of 10

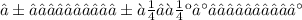
Median ↯
35, 48, 68, 73, 73, 81, 85, 88, 92

- n is the number of observations I.e. 9 in our case
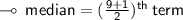
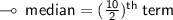
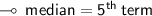

Mode ↯
35, 48, 68, 73, 73, 81, 85, 88, 92
- The most occurred number is 73 it has occurred 2 times. Hence, it is the mode!