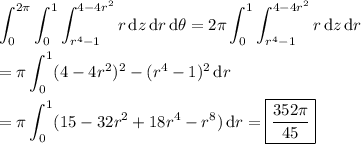In cylindrical coordinates, the equations of the surfaces become
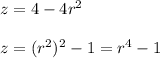
These surfaces intersect on the cylinder of radius 1 with cross sections parallel to the x,y-plane:
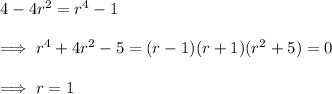
Then in cylindrical coordinates, the volume of the space bounded by these surfaces is