(b) The globe has a radius of 28.5 cm. Find the surface area of the globe. Round your answer to two decimal places. Use: 4\pi \: r ^(2) ​

Question

(b) The globe has a radius of 28.5 cm. Find the surface area of the globe. Round your answer to two decimal places. Use:


`4\pi \: r ^(2)`
​

Answer 1

`\boxed {\boxed {\sf 10,207.03 \ cm^2}}`

Explanation:

Surface area is the total area of the surface of an object. A globe is a sphere and the formula for calculating a sphere's surface area is:

`SA= 4 \pi r^2`

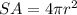
The radius (r) of the globe is 28.5 centimeters. Substitute this value into the formula.

• r= 28.5 cm

`SA= 4 \pi ( 28.5 \ cm)^2`

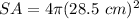
Solve the exponent. Multiply the number by itself.

• (28.5 cm)²= 28.5 cm * 28.5 cm = 812.25 cm²

`SA= 4 \pi (812.25 \ cm^2)`

Multiply 4, pi and 812.25 centimeters together.

`SA= 12.56637061 (812.25 \ cm^2)`

`SA= 10207.03453 \ cm^2`

Round to two decimal place or the hundredth place. The 4 in the thousandth place to the right (10207.03453) tells us to leave the 3 in the hundredth place (10207.03453).

`SA \approx 10207.03 \ cm^2`

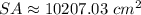
The surface area of the globe is approximately 10,207.03 centimeters squared.