Part A
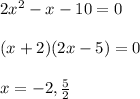
So, the x-intercepts are (-2,0) and (5/2, 0).
Part B
The vertex will be a minimum because the leading coefficient of the quadratic is positive, meaning the graph will open up.
The vertex has an x-coordinate that is the average of the roots, which in this case is 1/4.
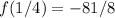
So, the coordinates of the vertex are
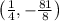
Part C
Plot the two x-intercepts and the vertex. Then, draw a parabola through these points that opens up.