Question

A certain forest covers an area of 5000 km². Suppose that each year this area decreases by 5%. What will the area be after 10 years?

Use the calculator provided and round your answer to the nearest square kilometer.

Answer 1

The forest will cover an area of approximately 2994 square kilometers after 10 years.

Explanation:

A forest covers an area of 5000 square kilometers. Each year, the area of the forest decreases by 5%. We want to determine its area after 10 years.

We can write an exponential function to represent the situation. The standard exponential function is given by:

`\displaystyle f(x) = a(r)^x`

Where a is the initial value, r is the rate, and x is the time that has passed (in this case in years).

Since the original area is 5000 square kilometers, let a = 5000.

Each year, the area decreases by 5%. In other words, for each subsequent year, the forest's area will be 100% - 5% = 95% = 0.95 of its previous year. Hence, r = 0.95.

Thus, our function is:

`\displaystyle f(x) = 5000(0.95)^x`

Then after 10 years (x = 10), the area will be:

`\displaystyle f(10) = 5000(0.95)^((10))= 2993.6846...\approx 2994\text{ km}^2`

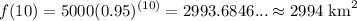
In conclusion, the forest will cover an area of approximately 2994 square kilometers after 10 years.