Answer:
Parallel:
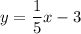
Perpendicular:
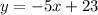
Explanation:
Hi there!
What we must know:
- Slope intercept form:
 where m is the slope and b is the y-intercept (the value of y when the line crosses the y-axis)
where m is the slope and b is the y-intercept (the value of y when the line crosses the y-axis) - Parallel lines always have the same slope
- Perpendicular lines always have slopes that are negative reciprocals (ex. 2 and -1/2, -6/7 and 7/6)
Finding the Parallel Line
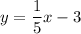
Given this equation, we can identify that its slope (m) is
 . Because parallel lines always have the same slope, the slope of the line we're currently solving for would be
. Because parallel lines always have the same slope, the slope of the line we're currently solving for would be
 as well. Plug this into
as well. Plug this into
 :
:
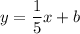
Now, to find the y-intercept, plug in the given point (5,-2) and solve for b:
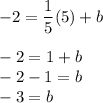
Therefore, the y-intercept is -3. Plug this back into
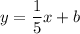 :
:
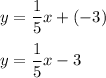
Our final equation is
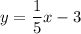 .
.
Finding the Perpendicular Line
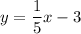
Again, the slope of this line is
 . The slopes of perpendicular lines are negative reciprocals, so the slope of the line we're solving for would be -5. Plug this into
. The slopes of perpendicular lines are negative reciprocals, so the slope of the line we're solving for would be -5. Plug this into
 :
:

To find the y-intercept, plug in the point (5,-2) and solve for b:
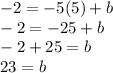
Therefore, the y-intercept is 23. Plug this back into
 :
:
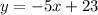
Our final equation is
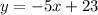 .
.
I hope this helps!