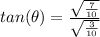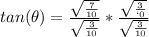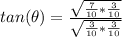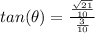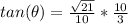Answer:

Explanation:
1. Approach
One is given the following information:

One can rewrite this as:

Also note, the problem says that the angle (
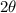 ) is found in the third quadrant.
) is found in the third quadrant.
Using the trigonometric identities (
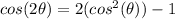 ) and (
) and (
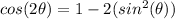 ) one can solve for the values of (
) one can solve for the values of (
 ) and (
) and (
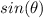 ). After doing so one can use another trigonometric identity (
). After doing so one can use another trigonometric identity (
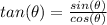 ). Substitute the given information into the ratio and simplify.
). Substitute the given information into the ratio and simplify.
2. Solve for
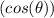
Use the following identity to solve for (
 ) when given the value (
) when given the value (
 ).
).
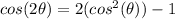
Substitute the given information in and solve for (
 ).
).
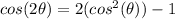
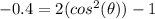
Inverse operations,
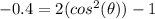
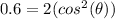
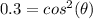
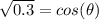
Since this angle is found in the third quadrant its value is actually:
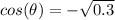
3. Solve for
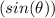
Use the other identity to solve for the value of (
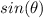 ) when given the value of (
) when given the value of (
 ).
).
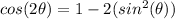
Substitute the given information in and solve for (
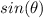 ).
).
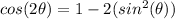
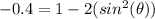
Inverse operations,
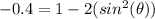

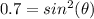
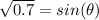
Since this angle is found in the third quadrant, its value is actually:

4. Solve for
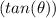
One can use the following identity to solve for
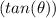 ;
;
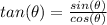
Substitute the values on just solved for and simplify,
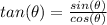
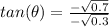

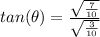
Rationalize the denominator,