Answer:
- When x = 2, y = 0
- When y = 5, x = 4
Explanation:
PART I: Value of y, when x = 2
Given information
x = 2
Given expression
2y - 5x + 10 = 0
Substitute values into the expression
2y - 5(2) + 10 = 0
Simplify by multiplication
2y - 10 + 10 = 0
2y = 0
Divide 2 on both sides
2y / 2 = 0 / 2
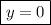
-----------------------------------------------------------------
PART II: Value of x, when y = 5
Given information
y = 5
Given expression
2y - 5x + 10 = 0
Substitute values into the expression
2(5) - 5x + 10 = 0
Simplify by multiplication
10 - 5x + 10 = 0
20 - 5x = 0
Add 5x on both sides
20 - 5x + 5x = 0 + 5x
20 = 5x
Divide 5 on both sides
20 / 5 = 5x / 5
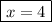
Hope this helps!! :)
Please let me know if you have any questions