Answer:
2. ☑ A
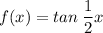
☐ B
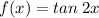
☐ C x =
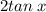
1. ☐ A
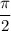
☐ B
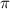
☑ C
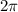
Step-by-step explanation:
![\displaystyle \boxed{f(x) = -cot\:((1)/(2)x - (\pi)/(2))} \\ \\ y = Acot(Bx - C) + D \\ \\ Vertical\:Shift \hookrightarrow D \\ Horisontal\:[Phase]\:Shift \hookrightarrow (C)/(B) \\ Wavelength\:[Period] \hookrightarrow (\pi)/(B) \\ Amplitude \hookrightarrow |A| \\ \\ Vertical\:Shift \hookrightarrow 0 \\ Horisontal\:[Phase]\:Shift \hookrightarrow (C)/(B) \hookrightarrow \boxed{\pi} \hookrightarrow ((\pi)/(2))/((1)/(2)) \\ Wavelength\:[Period] \hookrightarrow (\pi)/(B) \hookrightarrow \boxed{2\pi} \hookrightarrow (\pi)/((1)/(2)) \\ Amplitude \hookrightarrow N/A](https://img.qammunity.org/2022/formulas/mathematics/high-school/7o6yefvrog063665o52j0f8etg2q2igssr.png)
OR
![\displaystyle y = Atan(Bx - C) + D \\ \\ Vertical\:Shift \hookrightarrow D \\ Horisontal\:[Phase]\:Shift \hookrightarrow (C)/(B) \\ Wavelength\:[Period] \hookrightarrow (\pi)/(B) \\ Amplitude \hookrightarrow |A| \\ \\ Vertical\:Shift \hookrightarrow 0 \\ Horisontal\:[Phase]\:Shift \hookrightarrow 0 \\ Wavelength\:[Period] \hookrightarrow (\pi)/(B) \hookrightarrow \boxed{2\pi} \hookrightarrow (\pi)/((1)/(2)) \\ Amplitude \hookrightarrow N/A](https://img.qammunity.org/2022/formulas/mathematics/high-school/usl15cxieylfhxo6t51egbhiv8kt1o6x6b.png)
Here is all the information you will need. Now, what you need to know is that ALL tangent, secant, cosecant, and cotangent functions have NO amplitudes. Also, keep in mind that although this IS the tangent graph, if you plan on writing your equation as a function of cotangent, then there WILL be a horisontal shift, meaning that a C-term will be involved. As you can see, the photograph on the right displays the trigonometric graph of
 in which you need to replase "tangent" with "cotangent", then figure out the appropriate C-term that will make the graph horisontally shift and map onto the tangent graph [photograph on the left], accourding to the horisontal shift formula above. Also keep in mind that the −C gives you the OPPOCITE TERMS OF WHAT THEY REALLY ARE, so you must be careful with your calculations. So, between the two photographs, we can tell that the cotangent graph [photograph on the right] is shifted
in which you need to replase "tangent" with "cotangent", then figure out the appropriate C-term that will make the graph horisontally shift and map onto the tangent graph [photograph on the left], accourding to the horisontal shift formula above. Also keep in mind that the −C gives you the OPPOCITE TERMS OF WHAT THEY REALLY ARE, so you must be careful with your calculations. So, between the two photographs, we can tell that the cotangent graph [photograph on the right] is shifted
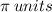 to the left, which means that in order to match the tangent graph [photograph on the left], we need to shift the graph FORWARD
to the left, which means that in order to match the tangent graph [photograph on the left], we need to shift the graph FORWARD
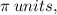 which means the C-term will be positive, and perfourming your calculations, you will arrive at
which means the C-term will be positive, and perfourming your calculations, you will arrive at
 but we are NOT YET DONE. Although we shifted the graph back into position, remember, cotangent is graphed in REVERCE, so we need to insert a negative in front of the amplitude term, and with that, the cotangent graph of the tangent graph, accourding to the horisontal shift, is
but we are NOT YET DONE. Although we shifted the graph back into position, remember, cotangent is graphed in REVERCE, so we need to insert a negative in front of the amplitude term, and with that, the cotangent graph of the tangent graph, accourding to the horisontal shift, is
 Now, with all that being said, we can move forward. To find the period in this case, you need to take a look at the distanse between each vertical asymptote. Now, accourding to this graph, the vertical asymptotes are
Now, with all that being said, we can move forward. To find the period in this case, you need to take a look at the distanse between each vertical asymptote. Now, accourding to this graph, the vertical asymptotes are
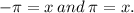 From here, you will then find the distanse between these asymptotes by simply perfourming the operation of Deduction, and doing that will give you
From here, you will then find the distanse between these asymptotes by simply perfourming the operation of Deduction, and doing that will give you
 So, the period of this function is
So, the period of this function is
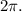 Now, you will instantly get the jist of the horisontal shift by looking at the above formula. Just keep in mind that the −C gives you the OPPOCITE TERMS OF WHAT THEY REALLY ARE, so you must pay cloce attention to what is given to you inside those parentheses. Finally, the midline is the centre of your graph, also known as the vertical shift, which in this case is at
Now, you will instantly get the jist of the horisontal shift by looking at the above formula. Just keep in mind that the −C gives you the OPPOCITE TERMS OF WHAT THEY REALLY ARE, so you must pay cloce attention to what is given to you inside those parentheses. Finally, the midline is the centre of your graph, also known as the vertical shift, which in this case is at
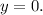
Well, that just about wraps it up. Now that everything has been explained, you should understand it better. If you still have questions, do not hesitate to comment any you have.
I am delighted to assist you at any time.