Answer:
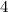
Step-by-step explanation:
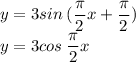
![\displaystyle y = Asin(Bx - C) + D \\ \\ Vertical\:Shift \hookrightarrow D \\ Horisontal\:[Phase]\:Shift \hookrightarrow (C)/(B) \\ Wavelength\:[Period] \hookrightarrow (2)/(B)\pi \\ Amplitude \hookrightarrow |A| \\ \\ Wavelength\:[Period] \hookrightarrow (2)/(B)\pi \hookrightarrow \boxed{4} \hookrightarrow (2)/((\pi)/(2))\pi](https://img.qammunity.org/2022/formulas/mathematics/high-school/sbcj56qiefk58tf9r9xhbxso5oif9t8of1.png)
OR
![\displaystyle y = Acos(Bx - C) + D \\ \\ Vertical\:Shift \hookrightarrow D \\ Horisontal\:[Phase]\:Shift \hookrightarrow (C)/(B) \\ Wavelength\:[Period] \hookrightarrow (2)/(B)\pi \\ Amplitude \hookrightarrow |A| \\ \\ Wavelength\:[Period] \hookrightarrow (2)/(B)\pi \hookrightarrow \boxed{4} \hookrightarrow (2)/((\pi)/(2))\pi](https://img.qammunity.org/2022/formulas/mathematics/high-school/4qzl0x0jwzb0iune75al15kdpgaeqvbv70.png)
You will need the above information to help you interpret the graph. So, sinse you ONLY have a graph to wourk with, you MUST figure the period out by using wavelengths. So, looking at where the graph hits
![\displaystyle [-5, 0],](https://img.qammunity.org/2022/formulas/mathematics/high-school/aon6rszygtw9cnjw3ybcvkjksbe14kwi2p.png) from there to
from there to
![\displaystyle [-1, 0],](https://img.qammunity.org/2022/formulas/mathematics/high-school/cwaloq3yoddznnxgwd2pvo5vs3otsc7ro1.png) they are obviously
they are obviously
 apart, telling you that the period of the graph is
apart, telling you that the period of the graph is
 Now, the amplitude is obvious to figure out because it is the A-term, but of cource, if you want to be certain it is the amplitude, look at the graph to see how low and high each crest extends beyond the midline. The midline is the centre of your graph, also known as the vertical shift, which in this case the centre is at
Now, the amplitude is obvious to figure out because it is the A-term, but of cource, if you want to be certain it is the amplitude, look at the graph to see how low and high each crest extends beyond the midline. The midline is the centre of your graph, also known as the vertical shift, which in this case the centre is at
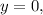 in which each crest is extended three units beyond the midline, hence, your amplitude. So, no matter how far the graph shifts vertically, the midline will ALWAYS follow.
in which each crest is extended three units beyond the midline, hence, your amplitude. So, no matter how far the graph shifts vertically, the midline will ALWAYS follow.
I am delighted to assist you at any time.