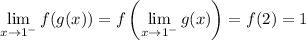Question

B) Lim[2f(x)-3g(x)]

Please do all if possible:) thank you!

Answer 1

Recall some limit properties…

• limits distribute over sums:

`\displaystyle \lim_(x\to c)(f(x)+g(x)) = \lim_(x\to c)f(x)+\lim_(x\to c)g(x)`

• limits distribute over products:

`\displaystyle \lim_(x\to c)(f(x)* g(x)) = \lim_(x\to c)f(x)*\lim_(x\to c)g(x)`

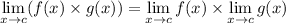
• limits distribute over quotients, provided that the denominator doesn't approach 0 :

`\displaystyle\lim_(x\to c)(f(x))/(g(x)) = (\displaystyle\lim_(x\to c)f(x))/(\displaystyle\lim_(x\to c)g(x))`

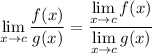
• if f(x) is continuous at x = c, then the limit "passes through" a composition:

`\displaystyle \lim_(x\to c)f(g(x)) = f\left(\lim_(x\to c)g(x)\right)`

# # #

(a) This limit is 2. At x = 2, we have f (2) = 1, but from either side of x = 2, we see f(x) approaching the point (2, 2). So

`\displaystyle \lim_(x\to 2)(f(x)+g(x)) = \lim_(x\to2)f(x)+\lim_(x\to2)g(x) = 2+0 = 2`

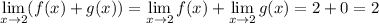
(b) This limit does not exist. We would have

`\displaystyle \lim_(x\to1)(2f(x)-3g(x)) = 2\lim_(x\to1)f(x)-3\lim_(x\to1)g(x)`

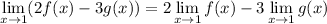
but g(x) approaches 2 from the left of x = 1, and g(x) approaches 1 from the right of x = 1. The one-sided limits don't match, so the two-sided limit doesn't exist.

(c) This limit is 0. It looks like f(x) passes through the origin, while g(x) ≈ 3/2 at x = 0. So

`\displaystyle\lim_(x\to0)f(x)g(x) = \lim_(x\to0)f(x)*\lim_(x\to0)g(x)=0*\frac32 = 0`

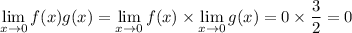
(d) This limit does not exist since

`\displaystyle \lim_(x\to-1)g(x)=0`

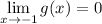
(e) This limit is 16. Nothing tricky here, just use the same property as in (c).

`\displaystyle\lim_(x\to2)x^3f(x) = \lim_(x\to2)x^3*\lim_(x\to2)f(x) = 8*2=16`

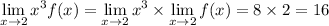
(f) This limit is 1. f(x) is continuous at x = 1, while g(x) approaches 2 from the left.

`\displaystyle\lim_(x\to1^-)f(g(x)) = f\left(\lim_(x\to1^-)g(x)\right) = f(2) = 1`