Question

Help please.
Simplify: 5i/ 3 + 2i

Answer 1

10+15i/13

Explanation:

This question is about complex numbers.

so,

in this type of questions we have to multiply this fraction by the conjugate of the denominator

conjugate of the denominator is 3-2i

1) 5i(3-2i)/(3+2i)(3-2i)

2) 15i-10i^2/9-4i^2 ( we know that i^2 = -1 )

3) 15i+10/9+4

4) 15i+10 / 13

Answer 2

The simplified form of
`(5i)/( (3+2i))` is
`(15i+10)/(13)`. Option A is the right choice.

To simplify
`(5i)/( (3+2i))`, we can multiply both the numerator and denominator by the conjugate of the denominator, which is 3−2i.

`(5i)/(3+2i) = (5i)/(3+2i) \cdot (3-2i)/(3-2i)`

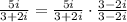
Using the distributive property and the fact that
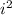`i^2` =−1, we can simplify the denominator as follows:

`(5i(3-2i))/((3+2i)(3-2i)) = (15i-10i^2)/(9-4i^2)`

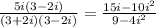
Substituting
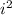`i^2` =−1, we get:

`(15i-10i^2)/(9-4i^2) = (15i+10)/(9+4) = (15i+10)/(13)`

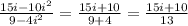
Option A is the right choice.