
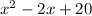
You are essentially asking at this point where is the quadratic function greater than 0. To get the interval find the roots. Between the roots the function is guaranteed to be LESS THAN zero. Everywhere else the function is greater than 0.

Use Vieta's rule to get the roots,
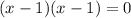
Both roots are the same so no value is less than 0 which means all values except at the point of root are strictly greater, the point of root is at
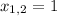 which means the set for which the quadratic function is greater than 0 is
which means the set for which the quadratic function is greater than 0 is
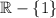 "all real numbers except 1".
"all real numbers except 1".
To convert this into interval notation use all numbers up to 1 but not including and all numbers after 1 but not including,
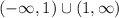
Hope this helps :)