Question

-3x^5y^7/6xy^8
PLEASE HELP

Answer 1

9514 1404 393

Answer:

-x^4/(2y)

Explanation:

Perhaps you want to simplify ...

`-(3x^5y^7)/(6xy^8)=-(3)/(6)x^(5-1)y^(7-8)=-(x^4y^(-1))/(2)=\boxed{-(x^4)/(2y)}`

__

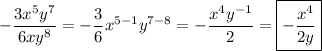
The applicable rules of exponents are ...

(a^b)/(a^c) = a^(b-c)

a^-b = 1/a^b

_____

Comment on notation

When writing a fraction in plain text, any denominator that includes an arithmetic operation must be enclosed in parentheses. Your given expression is properly written as ...

-3x^5y/(6xy^8)

Without the parentheses, the product xy^8 is in the numerator. This is demanded by the order of operations, which requires you evaluate your expression as (-3x^5y^7/6)·xy^8