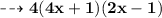1 Answer

Vladimir Sitnikov · Answer 1 · 2023-02-21T10:30:20+0000

Factorisation of a quadratic polynomial of the type ax² + bx + c where (a ≠ 1) .

To factorise ax² + bx + c, we have to find two numbers whose sum is equal to the coefficient of x and product is equal to the coefficient of x² and constant term.

Now,

Solution (1) :

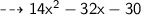
${ \qquad \sf { \dashrightarrow{ 14x^2 - 32x - 30 }}}$

${ \qquad \sf { \dashrightarrow{ 2(7x^2 - 16x - 15) }}}$

${ \qquad \sf { \dashrightarrow{ 2(7x^2 + 5x - 21x - 15) }}}$

${ \qquad \sf { \dashrightarrow{ 2[x(7x + 5) - 3(7x + 5)] }}}$

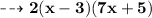
${ \qquad \bf { \dashrightarrow{ 2(x - 3)(7x + 5) }}}$

⠀

Solution (2) :

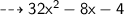
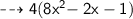
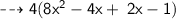
$\qquad \sf\dashrightarrow{ 32x^2 - 8x - 4 }$

${ \qquad \sf { \dashrightarrow{ 4(8x {}^(2) - \: }}} \sf2x - 1)$

${ \qquad \sf { \dashrightarrow{ 4(8x {}^(2) - 4x + \: \sf2x - 1)}}}$

${ \qquad \sf { \dashrightarrow{ 4[4x(2x-1) +1(2x-1)] }}}$

${ \qquad \bf { \dashrightarrow{ 4( 4x + 1)(2x-1) }}}$

Your comment on this question: