Question

Factor over the Reals

Answer 1

(x - 2y)³(1 - x+ 2y)(1 + x - 2y)

Explanation:

Given

(x - 2y)³ -
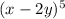`(x-2y)^(5)` ← factor out (x - 2y)³ from both terms

= (x - 2y)³(1 - (x - 2y)²) ← difference of squares which factors in general as

a² - b² = (a - b)(a + b) , then

1 - (x - 2y)²

= 1² - (x - 2y)²

= (1 - (x - 2y) )(1 + (x - 2y) )

= (1 - x + 2y)(1 + x - 2y)

Then

(x - 2y)³ -
`(x-2y)^(5)`

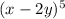
= (x - 2y)³(1 - x + 2y)(1 + x - 2y) ← in factored form