Question

Find the sum of a 22-term arithmetic sequence, where the first term is 7 and the last term is 240.

Answer 1

The sum of the arithmetic series is 2717.

Explanation:

The sum of an arithmetic sequence is given by:

`\displaystyle S = (k)/(2)\left( a + x_k\right)`

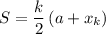
Where k is the number of terms, a is the initial term, and x_k is the last term.

There are 22 terms, the first term is 7, and the last term is 240. Hence, the sum is:

`\displaystyle \begin{aligned} S &= ((22))/(2)\left((7) + (240)} \\ \\ &= 11(247) \\ \\ &= 2717\end{aligned}`

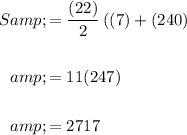
In conclusion, the sum of the arithmetic series is 2717.