Question

Given the function f(x) = -2c+cx-x^2, and f^-1(5) = -1, find c

Answer 1

`c=-2`

Explanation:

We are given the function:

`f(x) = -2c + cx - x^2`

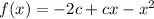
And that:

`\displaystyle f^(-1) (5) = -1`

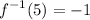
And we want to determine the value of c.

Recall that by definition of inverse functions:

`\displaystyle \text{If } f(a) = b, \text{ then } f^(-1)(b) = a`

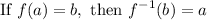
So, since f⁻¹(5) = -1, then f(-1) = 5.

Substitute:

`f(-1) = 5 = -2c + c(-1) - (-1)^2`

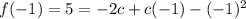
Simplify:

`5 = -2c - c - (1)`

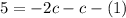
Combine like terms:

`6 = -3c`

And divide. Hence:

`c = -2`

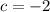
In conclusion, the value of c is -2.