Answer:
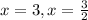
Explanation:

The zero product theorem will be used here to solve this non-linear problem.
means that in the original equation, each variable component must be set to zero.
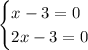
To solve this linear equation, we need to group all of the variable terms on one side of the equation and all of the constant terms on the other.
so, -3 will be moved to the right side
Notice that when a term moves from one side of the equation to the other, it changes sign.
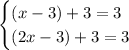
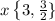
OAmalOHopeO