Answer:
Step-by-step explanation:
Important here is to know that due north is a 90 degree angle, due east is a 0 degree angle, and due south is a 270 degree angle. Then we find the x and y components of each part of this journey using the sin and cos of the angles multiplied by each magnitude:
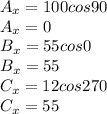
Add them all together to get the x component of the resultant vector, V:
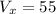
Do the same to find the y components of the part of this journey:
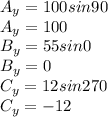
Add them together to get the y component of the resultant vector, V:
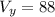
One thing of import to note is that both of these components are positive, so the resultant angle lies in QI.
We find the final magnitude:
 and, rounding to 2 sig dig's as needed:
and, rounding to 2 sig dig's as needed:
 1.0 × 10² m; now for the direction:
1.0 × 10² m; now for the direction:
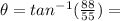 58°
58°